PAPER PLANES
Date: 2015
Client: Certain Measures
Team: Andrew Witt, Michelle Lee, Jim Peraino
Client: Certain Measures
Team: Andrew Witt, Michelle Lee, Jim Peraino
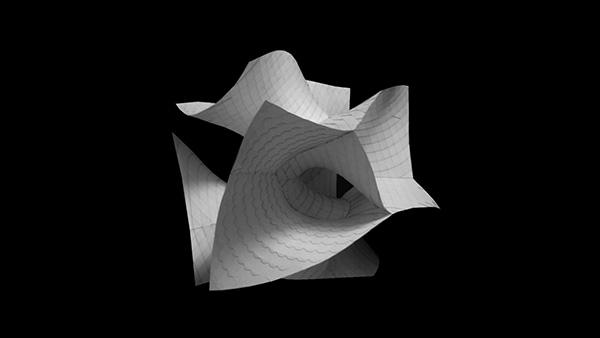
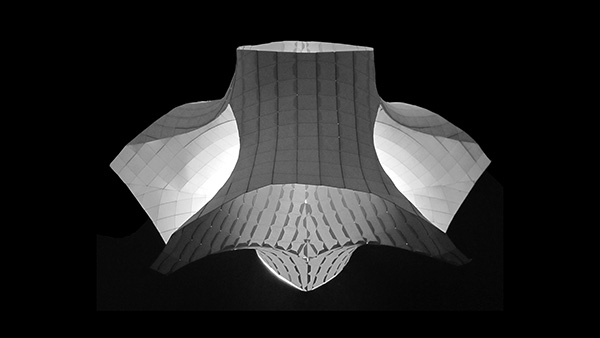
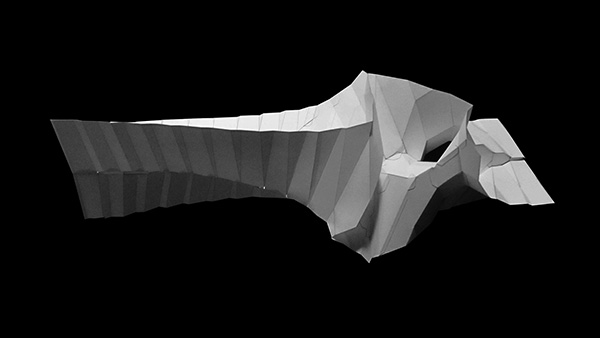
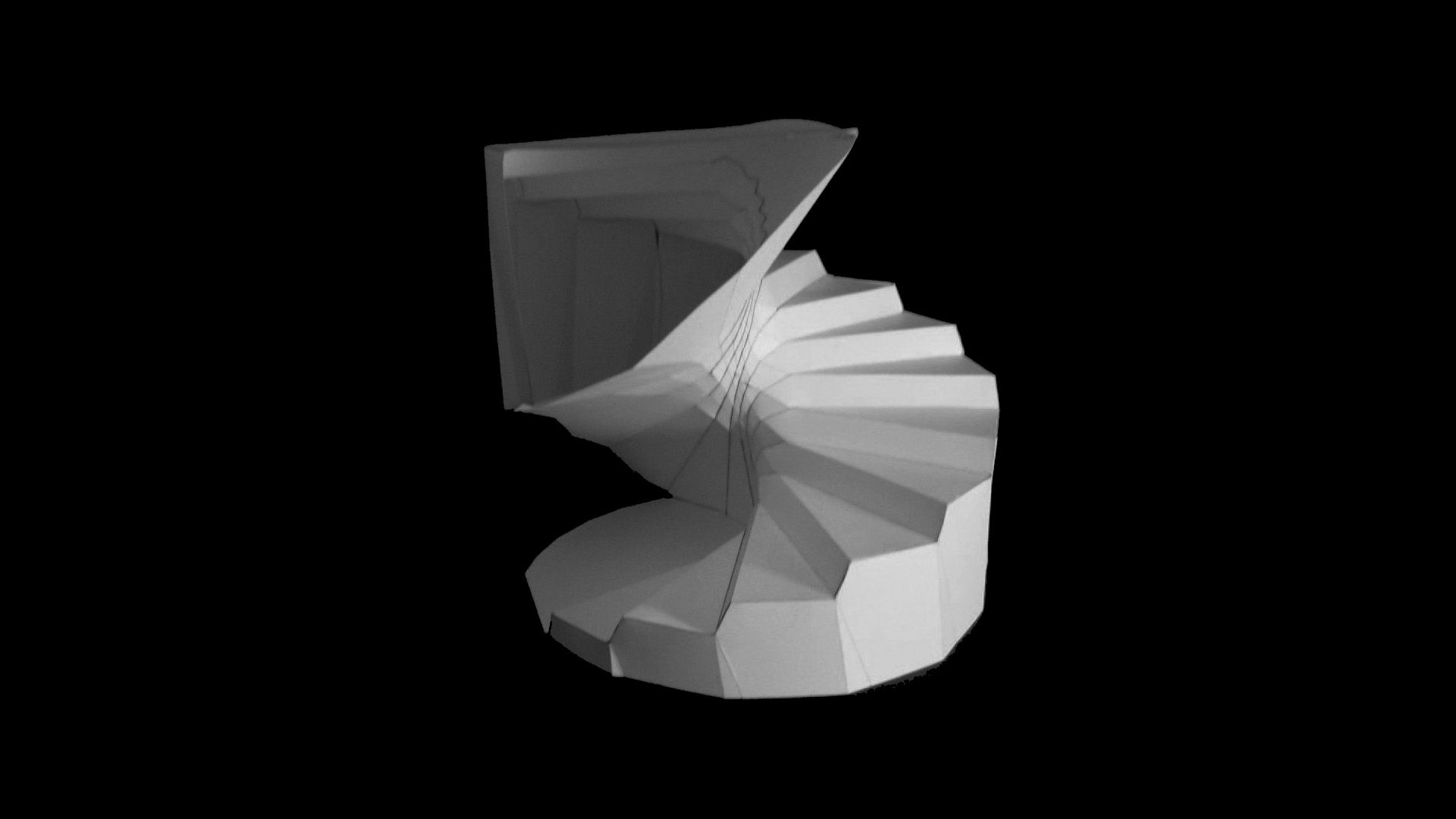
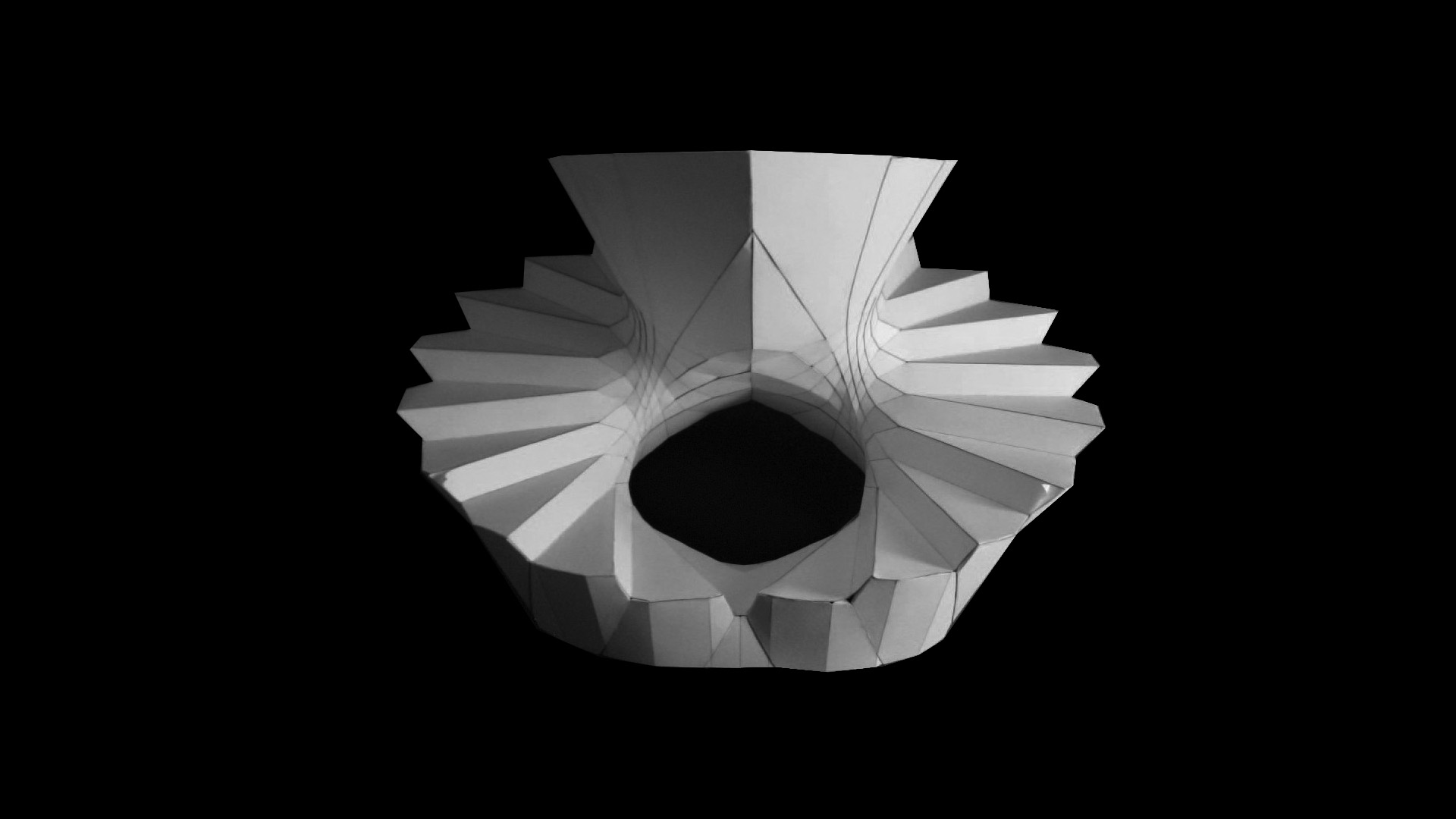
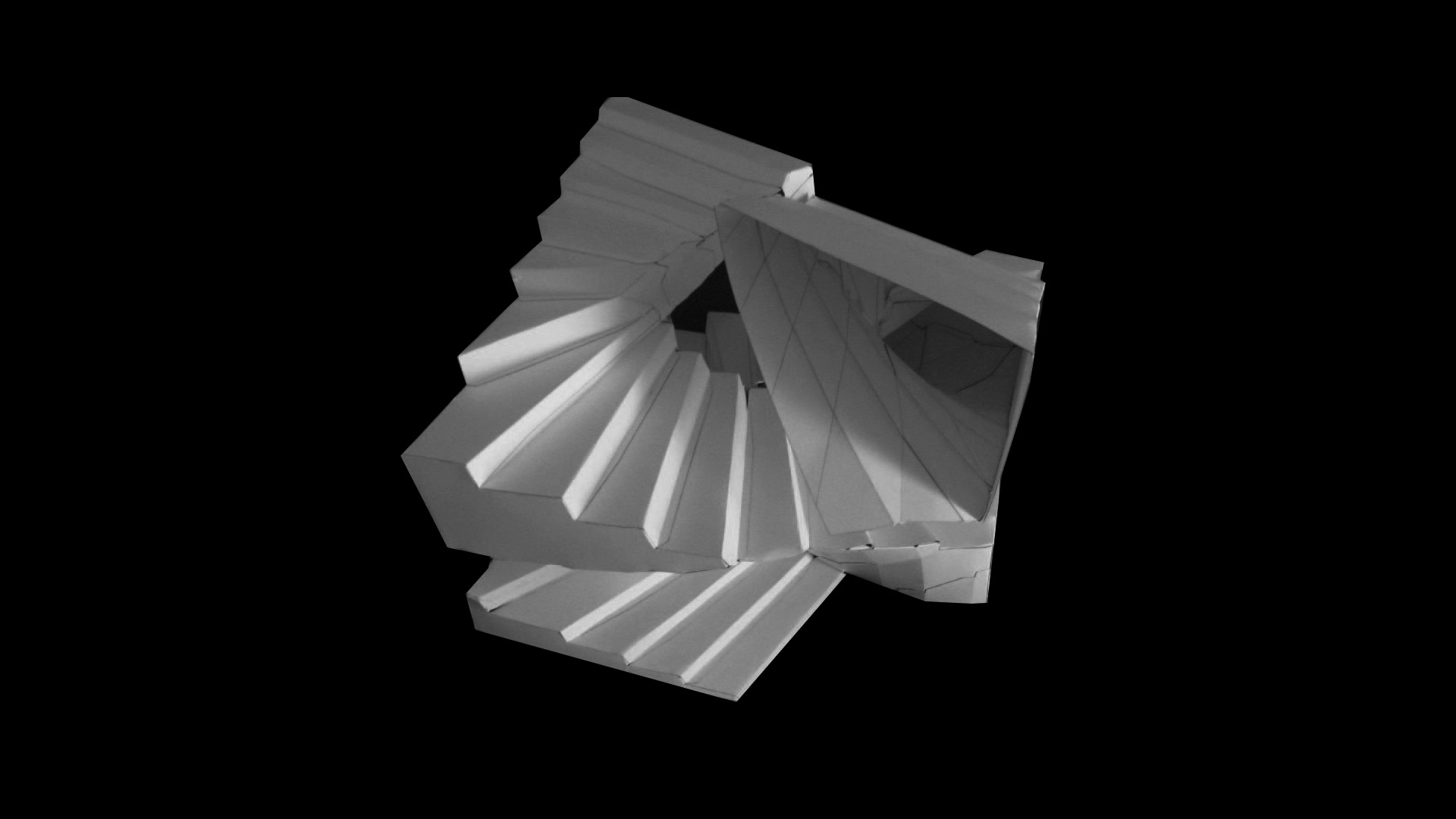
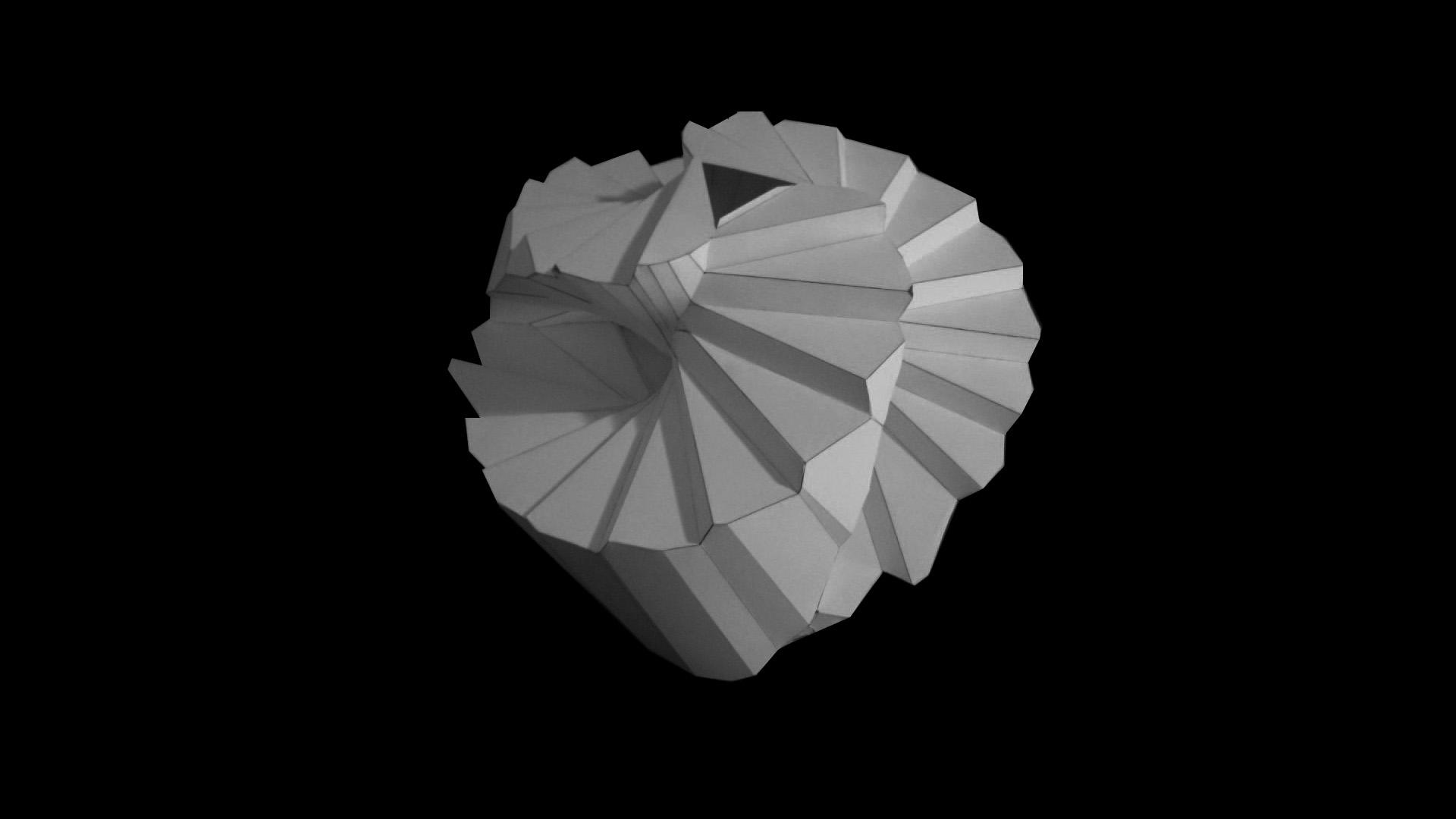
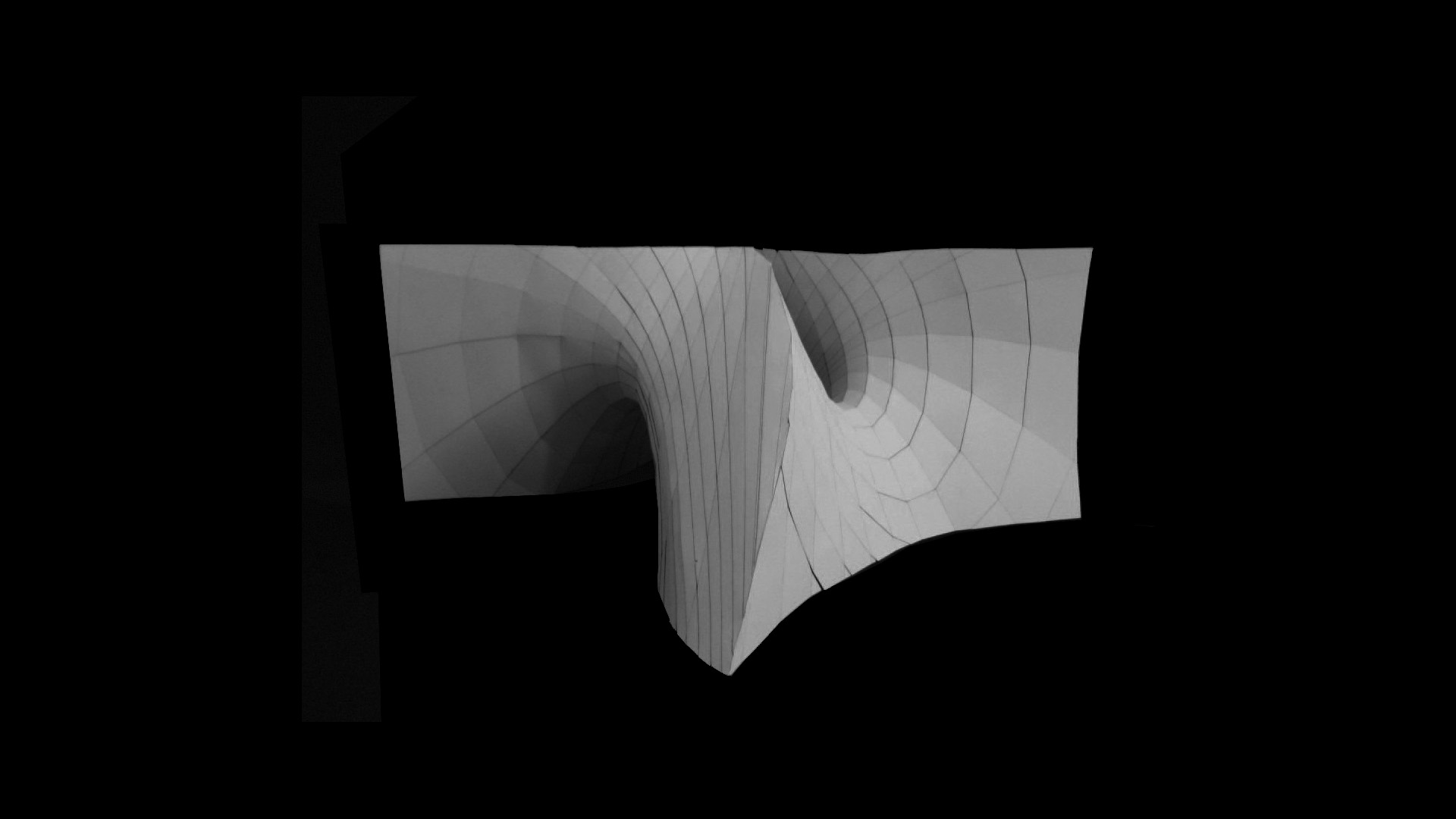
Paper Planes is body of research into complex surface approximations from planar quad meshes. Here we present two techniques: a quasi-zonohedral spanning surface, and medial surfaces.
The quasi-zonohedral surface is a method for constructing spanning surfaces with planar quads which transforms this edge curve into a textured and undulating interior. The method originates with a sampling of a number of points from the edge curve. The averages of the sequential partial sums of these perimeter points actually form the corners of concentric rings of planar quads. Thus any arbitrary boundary can be concentrically and iteratively patterned with planar quads in a simple and deductive way. Yet for such a direct method, it has a curious qualitative implication. In effect, the eccentricity of the boundary curve induces a ripple – a contorted standing wave – on the interior of the quad network, which you can see clearly in these models as they converge toward the center. The edge, in effect, has an echo.
The second method, the medial surface, begins from two or more circulation curves. These a sampling of these curves generates a series of mid-planes equidistant from pairwise sample points. Taken collectively, these define a planar-quad dominated mesh which is essentially the complement of the circulation space.
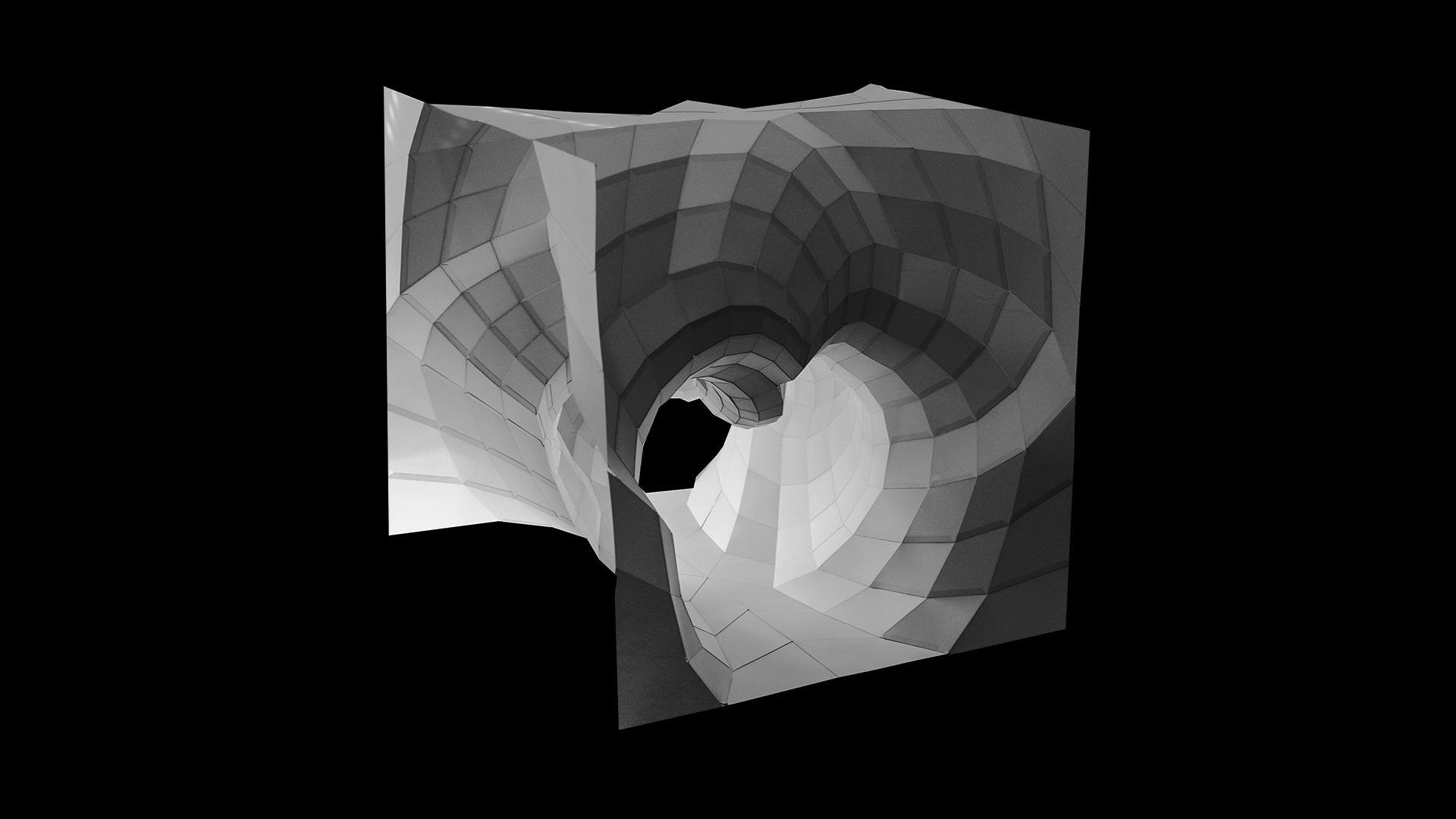
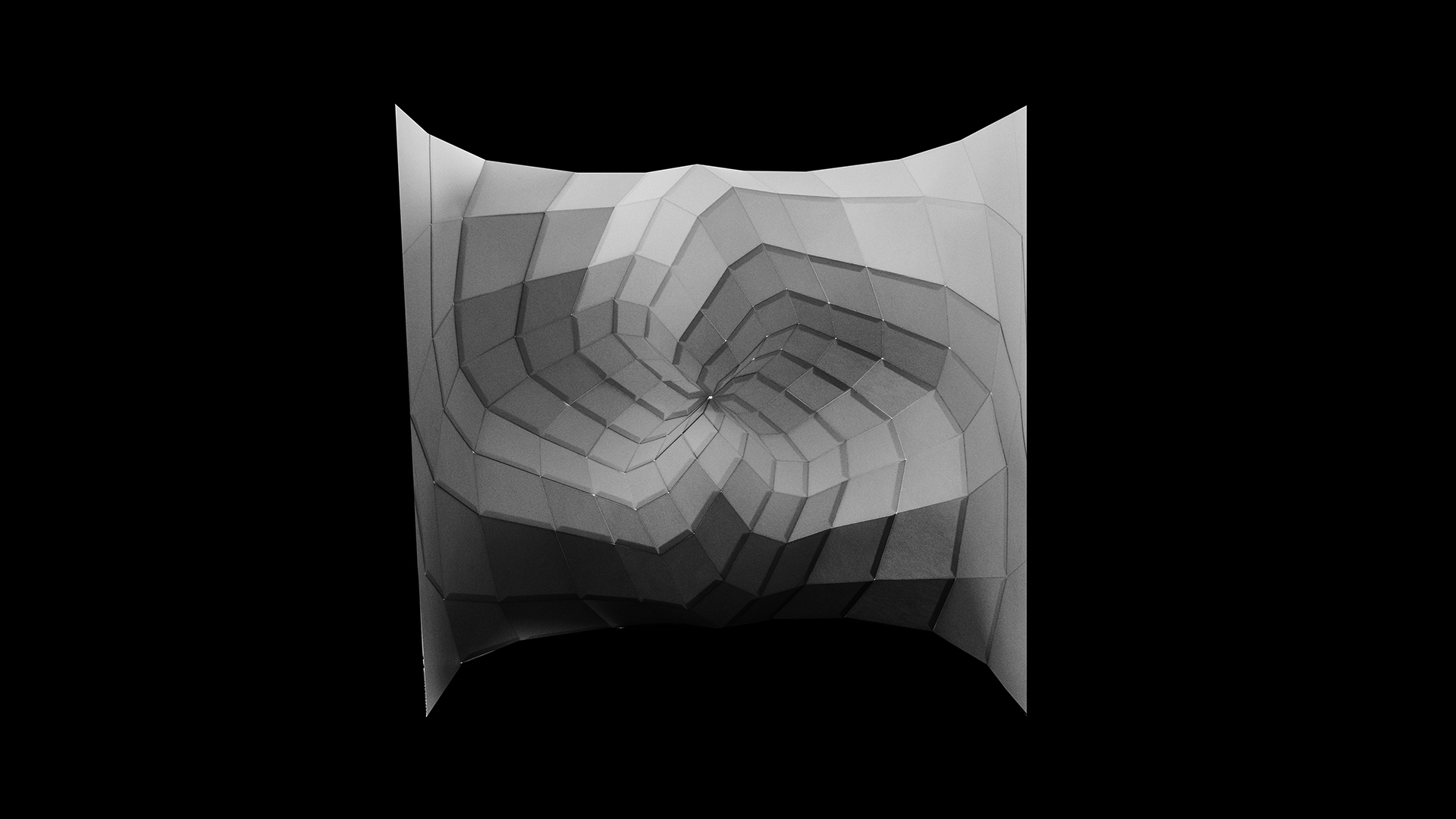
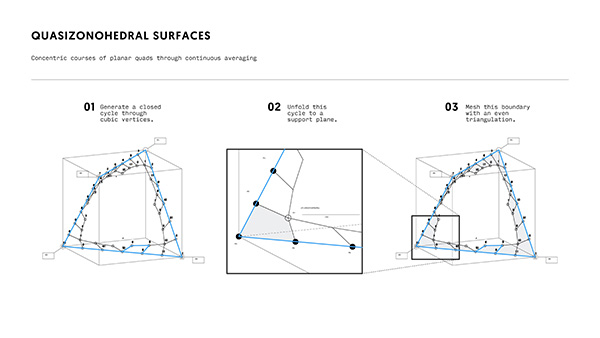

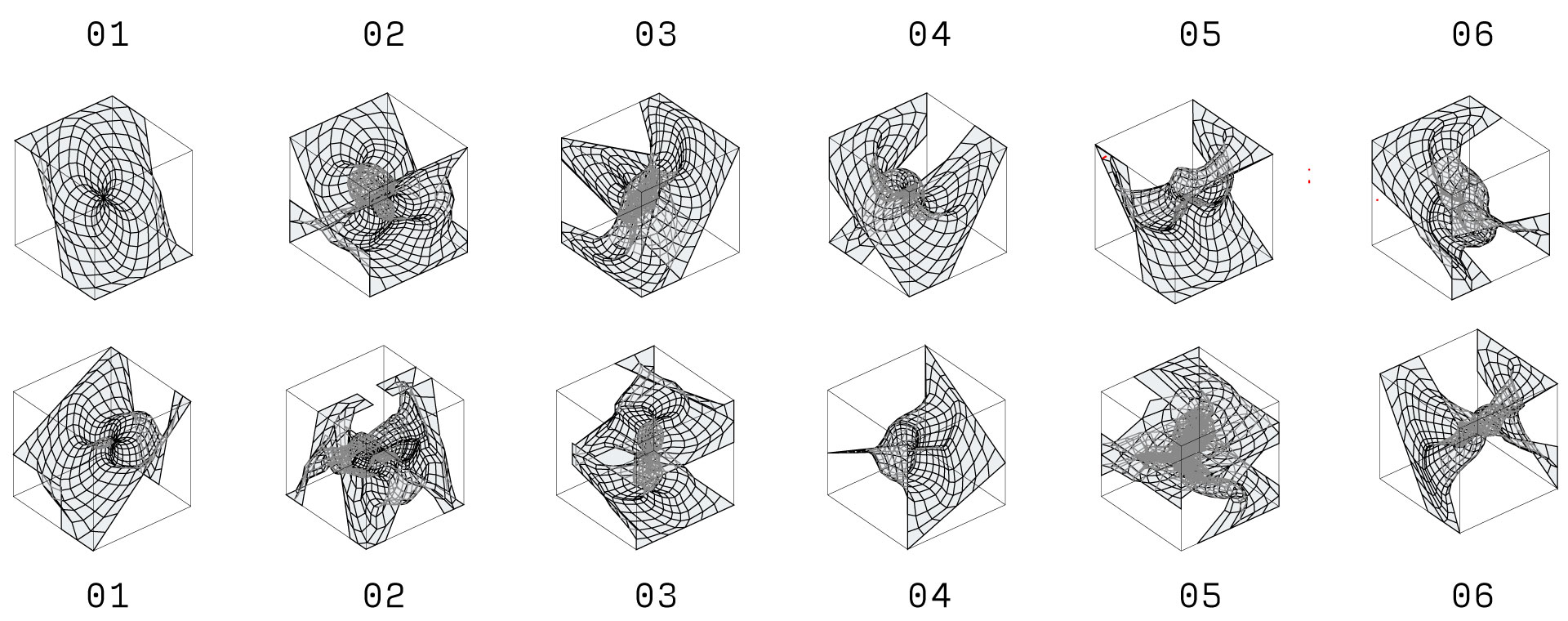
Quasizonohedral surfaces - Concentric PQ meshes